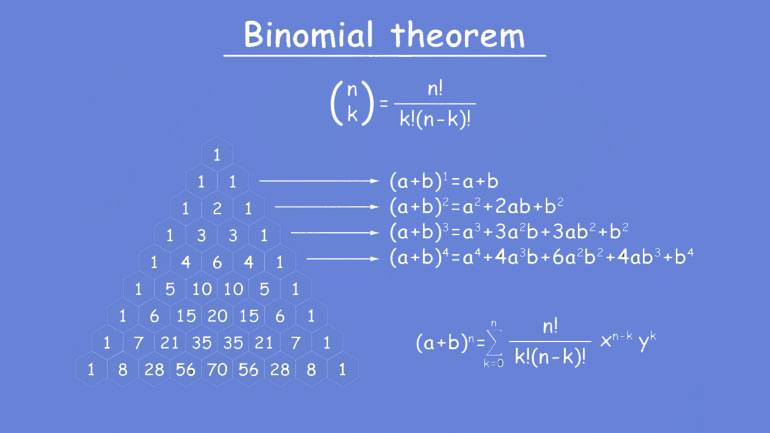
In mathematics, there are various theorems that we generally encounter such as the Pythagorean theorem, the Ceva’s theorem, and many more. Likewise, the binomial theorem is a type of theorem which helps to calculate or find the exponential value of an algebraic expression. Other algebras which are smaller in terms can be easily calculated with the help of algebraic formulas. The resultant or exponent value of the binomial theorem is always written in the form of a fraction of a negative number. A negative number can be defined as the type of number which is less than ‘0’. It is represented by the help of a – (negative) sign. A fraction is also a type of number which is written as a numerator/denominator. There are different terms related to the binomial theorem, one of them is known as a coefficient. The algebraic expression is written with the help of variables ‘x’ and ‘y’. In this article, we will try to cover some basic aspects of the binomial theorem such as the properties of this theorem, and some significant terms, and do a detailed analysis of it.
Some Important Terms Related to Binomial Theorem
As mentioned above, the binomial theorem is a type of theorem which helps to calculate or find the exponential value of an algebraic expression. There are various important terms such as general term, middle, term, etc. The following points analyze the significant terms related to the binomial theorem:
- The term which helps to represent or express all the terms of the binomial theorem is regarded as the general term. You will find a formula where x and y are the respective variables and r is denoted as the value which is less than the number of the binomial expansions.
- The term which is totally dependent on the value of n of the binomial theorem is known as the middle term. Here the value of n may be an even or odd number. Odd numbers are those numbers that cannot be classified in groups of two whereas even numbers are those numbers that are categorized as a group of two.
- The terms which are independent or not dependent on any other terms are regarded as independent terms.
How to Find the Binomial Coefficients Using the Pascal Triangle?
Binomial coefficients can be defined as the positive integers in the binomial theorem. Pascal’s triangle is regarded as the arrangement of these coefficients in a triangular form. This triangle is named after the name of great scientist, Blaise Pascal. Pascal’s triangles are generally used to find the coefficients of the binomial theorem but there are other uses as well such as this triangle is used in probability, calculus, and the theory of combinatorics. There are various features of the Pascal triangle. Some of them are mentioned below:
- The elements or numbers situated diagonally represent special kinds of numbers like natural, triangular, pentalobe, and tetrahedral.
- By adding those numbers (diagonal), we can get the value of Fibonacci numbers.
- If the second row consists of a prime number, then every other number will be divisible by that particular prime number.
Master Math from Math Online Classes
Mathematics is a very vital and significant subject for your future; thus the serious concern is to be looked after. For a very long time, teachers taught mathematics in a very boring way by providing statistics, examples, and formulas. Hence, Cuemath is there to help you as it is one of the leading personalized platforms that deal with math online classes. It also helps you by providing worksheets and puzzles math so that you study math in a fun way. There are various other facilities as well such as one-to-one live sessions, doubt clearing classes, and so on. Therefore, visit the Cuemath website to learn from their math online classes. Book a free session now.